The Laws of Acceleration

Abstract

Presented is a theory in fundamental theoretical physics that establishes the relationships between time, velocity, and the rate of acceleration for all material objects.  When properly formulated as given in this work, these relationships establish what appear to be two new natural laws of physics.  These laws, to be referred to as the Law of constant acceleration, and the Law of relative acceleration are in complete conformance with the principles of both, the time and energy theory, and the millennium theory of relativity.

The Laws of Acceleration

The Relationships Between Time, Velocity, and Rate of Acceleration

© 2001  Joseph A. Rybczyk

1. Introduction

The purpose of this paper is to identify the dual nature of constant acceleration and to establish the two laws that define its characteristics.  Whereas the first law deals with the constant aspect of such behavior, the second law deals with the relative aspect.  These two laws taken together establish all of the important relationships between the individual properties of time, velocity, and the rate of acceleration.  It is also noted that these laws conform to the principles of both, the time and energy theory¹, and the millennium theory of relativity², and represent the true relationships of the three stated properties for all objects undergoing constant acceleration.  We begin with the Law of constant acceleration, first introduced in the time and energy theory.

2. The Law of Constant Acceleration

As was previously established in the time and energy theory (* except for the identifying subscript added to the variable, a) the Law of constant acceleration is expressed as,

 

where, a_c, is the rate of constant acceleration, and v, is the resulting velocity over time interval, t`.  Time, t`, is then defined as,

 

where, t` represents time relative to the moving object, t, represents time in the stationary frame of reference, v, is the instantaneous velocity of the accelerating object, and, c, is the speed of light.

(* The subscript is needed to distinguish between the previously mentioned, two different aspects of acceleration.)

Specifically, the Law of constant acceleration states that the time interval during which acceleration takes place is directly proportional to the resulting velocity.  At first, this statement may seem contradictory because we are accustomed to thinking that, t`, becomes smaller as, v, increases.  While it is true that, t`, becomes smaller as a unit of time in relation to an increase in velocity, it is also true that the interval, t`, increases to show the total amount of time that has passed during the acceleration.  This concept is more easily understood if we revert for a moment to the classical, albeit incorrect, formula for constant acceleration,

 

In equation 3, because of the inherited (from the slowing of time) asymptotical nature of velocity, v, the rate of acceleration, a, cannot be constant.  In other words, as the increase in velocity, v, grows smaller, time, t, continues to increase at the original rate.  Once, t, is replaced with, t`, the increase in time is also asymptotical and the formula correctly defines a constant acceleration.

In view of the preceding analysis, it is apparent that equation 3 is invalid as a formula for showing constant acceleration.  From a practical standpoint, however, it is used for most everyday applications, because the asymptotic nature of velocity is insignificant at commonly experienced speeds.  From a purely technical standpoint, however, equation 3, in its intended use, has very limited application.  Consider, for example, an observer with a watch on his wrist.  Since the watch moves with the observer, it essentially has zero velocity relative to the observer and therefore always gives correct time relative to his frame of reference, even when he moves.  But, the moment the observer applies the time given by the watch to another object that is moving relative to him, the time is no longer correct.  The only truly correct time in such cases, is time t`.  In other words, the observer must convert time, t, given by the watch, to time, t`, given by equation 2.

With a little thought, it becomes evident that equation 1, is always correct, and equation 3 is nothing more than a special case of equation 1.  Whereas, equation 3 works satisfactorily at low velocities because the asymptotical nature of, v, is not yet apparent, equation 1, on the other hand, works at low velocities because at such velocities time, t`, essentially equals t.  Actually, t` = t, only when, v = 0.  At any other velocity it is smaller than t.  In plain words, time, t`, is always correct at any velocity, while time, t, is only correct at 0 velocity, and therefore equation 3 cannot be a correct formula for constant acceleration.  It turns out, however, that in spite of this, equation 3 has a very important role to play involving relative acceleration.  This role will be covered shortly under the heading, Law of Relative Acceleration.

3. Defining Acceleration

As innocent looking as equation 1 is, it raises serious questions about the proper definition of acceleration, and at the same time it provides us with yet another tool for testing the validity of relativistic principles.

Experimenting with the equation brings to light an apparent contradiction regarding the definition of acceleration.  Because of the asymptotical nature of velocity, the higher the rate of acceleration the more apparent the contradiction becomes.  For example, it will be found that if the rate of acceleration, a_c, reaches the extreme rate of .999c m/s, the velocity, v, reached in one second will not exceed .7075c m/s.  Moreover, when the rate of acceleration is increased beyond the given value, the achieved velocity will slowly reduce and never exceed .707c m/s regardless of how much higher the rate of acceleration is.  The question then becomes, which of the two values is the rate of acceleration?  If we go by the amount of energy used, we could say it is the value we assigned to, a_c.  On the other hand, we could also say that the rate of acceleration is the velocity reached at the end of one second.  Either can be construed as the correct answer, but for consistency, and to avoid confusion only one should be used.  Another solution would be to clearly define which value is being used in each particular case.  For the purpose of this paper, the value assigned to, a_c, is the rate of acceleration.

Although the case given above is extreme, the principle discussed is always present at any rate of acceleration.  To actually achieve in velocity, the rate of acceleration indicated by, a_c, in terms of a given distance traveled per unit of time, will always take more than a unit of that time.  This of course presents itself as a valid test of relativistic principles, because equation 1 predicts how much time is required for the resulting velocity to equal the rate of acceleration.

4. The Law of Relative Acceleration

Whereas the classical formula, equation 3, is incorrect for constant acceleration, it is correct for defining relative acceleration and is repeated here in modified form as the Law of relative acceleration.  Thus, where, a_r, is the rate of relative acceleration, and v, is the resulting velocity over time interval, t, equation 4 shows the correct relationship between these three values for all objects undergoing relative acceleration.

 

The use of equation 4 in this unintended application is supported by the following logic:  Since velocity, v, is asymptotic, as previously noted, and time interval, t, isn’t, it follows that the rate of acceleration, a_r, given by equation 4 will drop over time as the velocity increases.  In fact, as the velocity approaches c, the rate of acceleration approaches zero.  Whereas, it is obvious that, a_r, cannot be the rate of constant acceleration, it is equally obvious that it is the rate of relative acceleration between the accelerating object and an observer in the stationary frame of reference.  Moreover, the fact that equation 4 uses stationary time, t, and not moving frame time, t`, gives it a vital role to play involving both the rate of acceleration and the resultant velocity of an object undergoing acceleration.  Without equation 4, we have no way of using equation 1 in determining either the rate of constant acceleration, or the instantaneous velocity of an object under observation.  The reason is simple.  To use either equation, we need to be able to first determine the velocity or a change in velocity over an interval of time.  Because, t`, and, v, are interdependent variables, equation 1 cannot be used directly for this purpose.  However, since, v, has the same value in both, equation 1, and equation 4, and since, t, is not interdependent on, v, we can determine, v, using equation 4, and then substitute it into equation 1, along with t`, determined from, t, to find constant acceleration, a_c.  In fact, unlike the case with equation 1, in the case with equation 4, the relative acceleration, a_r, over a unit interval of time, t, always equals the instantaneous velocity, v.

5. Alternate Forms of the Two Laws

There are many relationships that may be developed through the integration of the two laws of acceleration.  Of these, perhaps the two most important are the alternate forms of the laws themselves.  The alternate form for constant acceleration is arrived at as follows:  From equation 4, we get,

 

the left side of which can be substituted in place of, v, into equation 1.  We can then also substitute the right side of equation 2 in place of, t`, in equation 1, to arrive at,

 

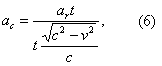
which simplifies to,

 

This is not only an alternate form of the Law of Constant Acceleration, but also shows clearly the relationship between constant acceleration and relative acceleration.  The following corollary of equation 7, of course, is the alternate form of the Law of Relative Acceleration: